Physical Quantities, Scalars, Vectors, Distance, Displacement, Speed and Velocity
The distance is a scalar quantity, while displacement is a vector quantity because the distance is determined by magnitude only, while displacement is determined by magnitude and direction, The direction of the wind affects the velocity of the plane and hence the time of the trip and therefore the amount of fuel consumed increases.
Types of physical quantities
To understand physical phenomena (which represent the greatest part of physics), it is necessary to deal with physical quantities and mathematical relationships, A physical quantity is any quantity that can be determined and has a measuring unit in our life, Each physical quantity is measured in a special measuring unit.
Examples of physical quantities are Mass, Time, Length, Speed, Force, Velocity, Displacement, and Acceleration.
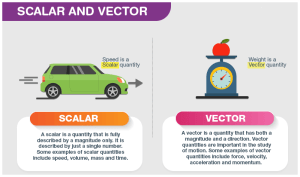
Types of physical quantities: Scalars, and Vectors.
Scalar physical quantities
To describe a scalar physical quantity. it is enough to know its magnitude only (numeric value and measuring unit), Scalar physical quantity is the physical quantity that has magnitude only and has no direction.
Examples:
- Mass: The measuring unit is Kilogram (kg).
- Time: The measuring unit is a Second or Hour.
- Length or Distance: The measuring unit is Metre (m).
- Speed: The measuring unit is m/sec. or km/h).
Length and time are scalar physical quantities because they have magnitude only and have no direction. All scalars are subject to algebraic mathematical operations related to numbers, So, they are added and subtracted if they have the same measurement units. The radius, area, and density are other examples of scalar physical quantities.
Vector physical quantities
To describe a vector physical quantity. it is necessary to know its magnitude (its numeric value and measuring unit) and also its direction. Vector physical quantity is the physical quantity that has magnitude and direction.
Examples:
- Force: The measuring unit is Newton.
- Velocity: measuring unit is m/sec. or km/h.
- Displacement: measuring unit is Metre (m).
- Acceleration: The measuring unit is m/sec² or km/h².
Acceleration and force are vector physical quantities because they have magnitude and direction. All vectors are subject to operations called vectors algebra. Vectors have great importance in different fields of physics, applied sciences like engineering. Understanding various physical phenomena such as gravity, movement of liquids and geometrical establishments depends basically on the main properties of vectors.
The difference between some similar physical quantities
There are some physical quantities that seem at first sight to be similar, but they have a great difference in their concept such as:
Distance and displacement
Example 1: When a car moves from position (A) to position (B). The length of the curved line gives the distance covered by the car. The straight line that starts from point (A) and ends at point (B) in the direction AB gives the displacement of the car. (The shortest length between (A) and (B) in direction AB [in the Eastward direction].
Example 2: A person wants to make a trip by his car from Caire (starting point to Tania (endpoint). The distance of the trip depends on the path that the car takes til may take place in two ways. If the trip path is:
- (Cairo → Benha→ Tanta), The distance covered= 45+ 60= 105 km.
- (Cairo→ Zagazig→ Tanta), The distance covered= 80+ 85= 165 km.
You notice the difference in the distance between Cairo and Tanta (although the two cities remain constant), this difference in distance depends on the trip path. The shortest straight line that starts from Cairo and ends at Tanta represents the displacement of Tanta from Cairo which does not change. i.e. The displacement= 93 km in the Northern west direction “Vector quantity“.
Example 3: When a body moves in a straight line from (A) to (B) covering 50 metres, then it returns back to (A). Therefore: The distance covered from (A) to (B) then to (A)= 50+ 50= 100 m. The displacement from (A) to (B) then to (A) = Zero, because the body returns back to its starting point (A).
The distance= The displacement (numerically) when the body moves in a straight line in one direction, this means that: The distance covered from (A) to (B) = 50 m. The displacement from (A) to (B) = 50 m in direction AB.
Distance
Distance (d) is the actual length of the path that a moving object covers from the starting point to the ending point. When the distance covered by an object equals 30 km. This means that the actual length of the path that a moving object covers from the starting point to the ending point equals 30 km. Distance is a scalar physical quantity because it is identified by a magnitude only. Measuring unit: Metre or kilometre.
Displacement
Displacement is the distance covered at a certain direction from the primary position of movement towards its final position. Amount of displacement is the length of the shortest straight line between two positions (primary position and final position). Measuring unit: Metre or kilometre.
When the displacement of an object equals 200 km in the west direction, This means that the distance covered in the west direction from the primary position of movement towards its final position equals 200 km. Displacement is a vector physical quantity because it is identified by magnitude and direction.
The two equal displacements must have the same magnitude and the same direction. The direction is determined from the starting point of the movement towards the final point.
When the amount of displacement is less than the distance covered, so, the object moves in a curved path (or any path that doesn’t represent a straight line). When the displacement of 2 different objects is equal. so, they have the same magnitude and move in the same direction.
The displacement of a moving object equals zero. When the object returns to its primary position of movement, where the final position of movement is the same of its primary position.
Problems
A rubber ball fell downward from a height equal 2 metres from the ground, then returned upward 1 metre, then fell down again to rest on the ground. Calculate distance and displacement.
Solution
1. Distance = 2+ 1+ 1= 4 metres.
2. Displacement = 2 metres downward
A car starts motion from point (A) and covers 30 metres northward to point (B) within 30 seconds, then 60 metres eastward to point (C) within 20 seconds, and then 30 metres southward to point (D) within 10 seconds. Find:
- Total distance covered by this car.
- Total time that car took to cover this distance.
- Displacement and what is its direction.
Solution
- Total distance= 30+ 60+ 30 = 120 m
- Total time= 30+ 20+ 10= 60 sec.
- Displacement= 60 m in the direction (AD).
Speed and velocity
Average speed is the total distance covered by the object in one second (a unit time). Speed can be identified by its magnitude only. It is a scalar physical quantity.
Average velocity is the displacement covered by the object in one second (a unit time) or It is the rate of change of displacement. Speed can be identified by its magnitude only. Velocity can be identified by its magnitude and direction. It is Vector physical quantity.
When a body covered 50 m northward direction in 5 see. This means that the velocity of this body equals 10 m/sec in the northward direction. The measuring units of velocity are m/see or km/h [The same measuring units of speed). When an object moves, where its starting point is the ending point, its velocity equals zero. Because the displacement of this object is zero.
The velocity is the speed in a given direction (When the body moves in a straight line in one direction). Velocity is a vector physical quantity and to determine it accurately, it is necessary to identify its magnitude and direction.
Such as when we say that the predator (Cheetah) is one of the fastest wild animals. its speed is about 27 m/sec, but if we want to represent its velocity, we say that its velocity is 27 m/sec in a certain direction. We can say that Cheetah’s velocity = 27 m/sec in the westward direction.
A body covered 30 metres northward within 30 seconds, then 40 metres eastward within 20 seconds and stopped at a point about 50 metres from the starting point. Calculate:
- Total distance moved by the body.
- Average speed.
- Displacement.
- Average velocity.
Solution
- Total distance= 30 + 40= 70 m.
- Average speed= Total distance/ Total time= (30 + 40) /(30+ 20) = 1.4 m/sec.
- Displacement = 50 m in the northern east direction.
- Average velocity= 50/ 50= 1 m/sec. in the northern east direction.
Importance of wind velocity for flights
The movement of wind arises from the difference in air pressure in different regions above the Earth’s surface. The movement of the Earth around itself from west to east affects the direction of the wind, which affects the value of the velocity of the plane, So. Pilots take in consideration the velocity of the wind.
When the plane takes off in the same direction of wind from city (1) northeast direction to city (2). The value of its velocity increases, so, the time of the trip decreases, and therefore the amount of fuel consumed decreases
When the plane takes off in the opposite direction of wind from city (2) southwest direction to city (1). The value of its velocity decreases due to the resistance of the wind to the movement of the plane, so the time of the trip increases, and therefore the amount of fuel consumed increases.
The amount of consumed fuel by a plane flies between two cities differs according to the wind direction. because when the plane flies against the wind direction, its velocity decreases and it consumes more fuel and takes more time than when it flies in the same direction of the wind direction.
You can subscribe to Science Online on YouTube from this link: Science Online
You can download the Science Online application on Google Play from this link: Science Online Apps on Google Play
Acceleration types, units, importance & Graphic representation of moving in a straight line
Motion in one direction, Types of Speed, Average Speed & Relative Speed
Types of motion, Relative motion, Applications of Mechanical waves & Electromagnetic waves
Role of waves in transferring energy, Wave Motion, Transverse waves & Longitudinal waves
Laws of circular motion (Centripetal Acceleration, Tangential linear Velocity & Centripetal Force)
Scalar Quantities, Vector Quantities & Finding resultant of two perpendicular forces