Kirchhoff’s first law, Kirchhoff’s second law and how to solve problems of Kirchhoff’s laws

Some electric circuits consist of many branches and are too complicated to be managed by Ohm’s law because the electric current passing through them is different in each branch, For such cases, a German scientist called Kirchhoff set two relations called Kirchhoff’s laws which enable one to analyze arbitrary circuits.

Kirchhoff’s first law: based on conservation of electric charge

The electric current through a metallic conductor is a stream of negative free electrons (electric charges) flowing from one point to another, These charges do not accumulate at any point in the circuit but they move through the circuit continuously & the conductor is not charged during the flow of the electric current.

According to this Kirchhoff has deduced that the sum of charges flowing into the node is equal to the sum of charges flowing out of that node and formulated his first law ” Kirchhoff’s current law ” as follows:

Kirchhoff’s first law: At any node (junction) in an electrical circuit, the sum of currents flowing into the node is the sum of currents flowing out of that node, or the algebraic sum of the electric currents at a point (a node) in a closed circuit equals zero.

The mathematical relation for Kirchhoff’s first law 

Sum of currents flowing into the point (A) = Sum of currents flowing out of point (A)

∑ I_(in) = ∑ I_(out)

At the junction (node), The current flowing into the point and that flowing out of it have a positive sign (+).

I₁ + I₂ = I₃ + I₄

The algebraic sum of currents at the junction in a closed circuit = zero 

∑ I = 0 

At the junction (node), The current flowing into the point has a positive sign (+), The current flowing out of the point has a negative sign (−).

I₁ + I₂ − I₃ −  I₄ = 0

Kirchhoff’s first law is used in the circuits that include resistors connected in parallel due to the presence of junctions for currents distribution.

Kirchhoff’s first law is an application of the law of conservation of charge (the quantity of charge flowing into a node is equal to the quantity of charge flowing out of that node) because the electric current intensity in (Amperes) equals the quantity of electric charges in (Coulomb) crossing a cross-section area in one second.

Kirchhoff’s second law: based on conservation of energy 

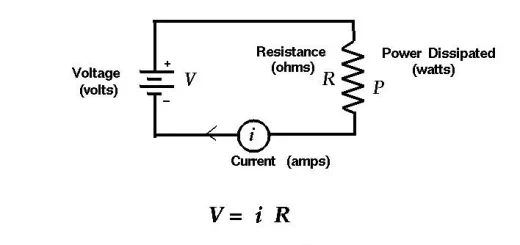
Potential difference (V) expresses the work done or the energy required to transfer a unit electric charge across a component in the circuit, It is measured in Volt, It is calculated from the relation: V = I R, Where: R is the resistance of the part in the circuit at which the potential difference is required to be calculated at its ends.

Electromotive force (V_B) expresses the work done or the energy required to transfer a unit electric charge once round the whole circuit, It is measured in Volt, It is calculated from the relation: V_B = I (R‾ + r), Where: (R‾) is the total external resistance, (r) is the internal resistance of the source.

This is formulated by Kirchhoff in his second law (Kirchhoff’s voltage law) as follows:

Kirchhoff’s second law: The algebraic sum of the electromotive forces in any closed loop is equivalent to the algebraic sum of the potential differences within that loop, or the algebraic sum of the potential differences in any closed loop equals zero.

When solving problems of Kirchhoff’s second law, the direction of every closed path must be determined clockwise or counterclockwise.

The mathematical relation for Kirchhoff’s second law 

The algebraic sum of the electromotive forces = The algebraic sum of the potential differences.

∑ V_B = ∑ I R

(V_B)₁ + (V_B)₂ = I R₁ + I R₂

The algebraic sum of the potential difference in the electric circuit = zero

∑ V = 0

(V_B)₁ + (V_B)₂ − I R₁ − I R₂ = 0

Kirchhoff’s second law can be applied to more than one closed loop, Kirchhoff’s second law is considered an application for the law of conservation of energy, when applying Kirchhoff’s second law on a closed loop, the following sign rule should be respected:

If the mathematical formula: (∑ V_B = ∑ I R)

If the proposed direction from the negative pole to the positive pole inside the source (battery) then the value of the emf for this source is positive, If the proposed direction from the positive pole to the negative pole inside the source (battery) then the value of the emf for this source is negative.

If the resistors are connected together in parallel or in series, it is preferable to find their equivalent resistance before applying Kirchhoff’s laws.

If the proposed direction is the same as that of the current passing in a certain resistance, then the value of the potential difference between the ends of the resistance is positive, If the proposed direction is opposite to that of the current passing in a certain resistance, then the value of the potential difference between the ends of the resistance is negative.

If the mathematical formula: ∑ V = 0

If the proposed direction from the negative pole to the positive pole inside the source (battery) then the emf for this source is positive, If the proposed direction from the positive pole to the negative pole inside the source (battery) then the emf for this source is negative.

If the proposed direction is the same as that of the current passing in a certain resistance then the potential difference between the ends of that resistance is negative, If the proposed direction is opposite to that of the current passing in a certain resistance then the potential difference between the ends of that resistance is positive.

How to solve problems of Kirchhoff’s laws

For the electric circuit to calculate the electric current intensity passing through each resistance, we go through the following steps:

1. Determine the number of the unknown quantities required to be calculated, Propose certain direction for each unknown current (These directions are not all necessarily to be correct), Determine direction for each closed path randomly (clockwise or anti-clockwise).
2. Apply Kirchhoff’s first law at a junction for the current once such that: Sum of input currents = Sum of output currents, Thus you obtained the first equation.
3. Choose a closed loop and apply Kirchhoff’s second law such that: Algebraic sum of emf = Algebraic sum of potential differences, where the sign rule should be respected.
4. Repeat the previous step for many loops till the number of equations equals the number of unknown values.
5. Solve equations to obtain the unknown values which are I₁, I₂, and I₃.
6. If the calculated value for the current was positive: then the correct direction is the same as that of the proposed direction from the beginning, Negative: then the correct direction is opposite to that of the proposed direction from the beginning.

Ohm’s Law for the closed circuit, Relation between emf (VB) of an electric cell & voltage across its poles