Applications on the pressure at a point (Connected vessels, U-shaped tube & Mercuric barometer)
Atmospheric pressure is the weight of a column of air above the Earth’s surface per unit, As we go up, the height of this column decreases, so, does the pressure, At the eardrum, the external pressure must be balanced out by an internal pressure When the external pressure decreases, we feel tense at the ear drum.
Since the internal pressure pushes it outwards, this can be compensated by adjusting the amount of air in the eustachian tube by swallowing and chewing gum to reduce the pressure on the eardrum.
Applications on the pressure
There are many applications for the pressure at a point inside the liquid such as Connected vessels, U-shaped tube, Mercuric barometers, and Manometer.
Connected vessels
A container consists of many vessels of different geometrical shapes connected together at its bases, The pressure at all points in the same horizontal plane in a homogeneous liquid is the same, The level of liquid at all parts is the same regardless of its geometrical shape on one condition that their bases are in the horizontal plane.
U-shaped tube
Structure: It consists of a U-shaped tube where the pressure at all points in the same horizontal plane in a homogeneous liquid is the same, It is used to compare the density of two liquids, It is used to determine the density of a liquid by knowing the density of another liquid, It is used to determine the relative density of a liquid that does not mix with water (immiscible liquid).
Determination of the density of oil by knowing the density of water using the U-shaped tube:
Put a suitable amount of water in the U-shaped tube so that the level of water is the same on both sides, Pour oil gently on one of the sides so that a separating surface is formed between oil and water as they are immiscible liquids, Measure the height of water (hw) and that of oil (ho) above the separating surface when they are in equilibrium.
The density of oil can be determined as follows:
P1 = P2
Pa + ho g ρo = Pa + hw g ρw
ho ρo = hw ρw
ρo / ρw = hw / ho
Where: (ρo / ρw) is the relative density of oil.
Density of oil can be determined using the relation by knowing the density of water :
ρo = hw ρw ÷ ho
The height of a liquid in the tube is inversely proportional to its density h ∝ 1/ρ, The radius of the tube or the cross-sectional area of two branches does not affect the height of the two liquids in the branches, we can apply the following relation ho ρo = hw ρw, in U-shaped tube with different diameter.
In the case of two miscible liquids, they can be separated by a third liquid that is immiscible with each of them like mercury which can separate between water and alcohol.
In the case of the balance in a U-shaped tube:
Balance between two liquids, h1 ρ1 = h2 ρ2
Balance between more than two liquids, h1 ρ1 = h2 ρ2 + h3 ρ3
Where the radius of the tube does not affect the liquid height on the sides of the tube.
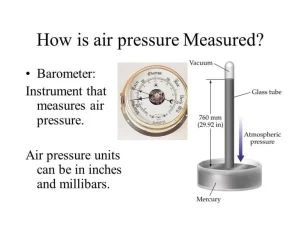
Mercuric barometer
A scientist named Torricelli invented the mercuric barometer to measure the atmospheric pressure, A glass tube of length 1 meter and opened at one of its ends is completely filled with mercury then turned upside down in a tank of mercury.
The level of mercury goes down to a certain limit that measured 0.76 m from the surface of mercury in the tank, The space above the column of mercury in the tube is a vacuum and called the Torricelli vacuum, Torricelli vacuum is the space above the mercury inside the tube of the mercuric barometer that is evacuated except a few mercury vapor.
The pressure at all points in the same horizontal plane in a homogeneous liquid is the same, the atmospheric pressure is equivalent to the weight of a column of mercury whose height is 0.76 m and cross-sectional area is 1 m² at 0° C at sea level.
The atmospheric pressure (Pa) is the weight of the air column whose base is the unit area and its height extends from the sea level to the top of the atmosphere, or it is the air pressure at sea level which is equivalent to the pressure due to weight of mercury column of height 0.76 m and of base area 1 m² at 0° C.
When the atmospheric pressure = 1.013 × 105 N/m², It means that the weight of the air column whose base is the unit area and its height extends from the sea level to the top of the atmosphere = 1.013 × 105 N.
Mercury is used as a barometric substance because the density of mercury is high, so, its height becomes suitable to the length of the barometer tube where: (h ∝ 1/ρ), Also no pressure in Torricelli vacuum because no mercury vapor in normal temperature.
Water can not be used instead of mercury in the barometer because the density of water is much less than that of mercury, so if water is used then the length of the tube of the barometer must be greater than 10 meters.
Mercury height in the barometer is not affected by the cross-sectional area of the barometric tube, Based on the relation (P = ρgh) height of mercury in the barometer depends on the mercury density only and not on the cross-section area of the tube.
The reading of the mercuric barometer depends on the atmospheric pressure acting on the surface of mercury in the mercury tank which changes by changing temperature and height from the sea level.
The reading of the mercuric barometer does not depend on the length of the tube, the volume of the Torricelli vacuum, The length of the submerged part of the tube under the mercury surface, Torricelli vacuum disappears in the barometric tube when the vertical height of the tube from the mercury level is less than or equals 76 cm.
Uses of the mercuric barometer
Determination of the atmospheric pressure.
Determination of the height of a mountain or a building.
Determination of the density of air.
Determination of the atmospheric pressure
Atmospheric pressure = Pressure at point (A)
Pa = PA = ρgh
Where: ρ is the density of mercury and equals 13595 kg/m³, g is the acceleration due to gravity and equals 9.81 m/s², h is the height of mercury in the barometric tube and equals 0.76 m, Pa = 13595 × 9.8 × 0.76 = 1.013 × 105 N/m²
Determination of the height of a mountain or a determination of the density of air
When measuring the height of the mercury column in a barometer at the bottom of a mountain (h1) and then at the top of that mountain (h2), then the difference in pressure measured by the barometer = difference in the atmospheric pressure.
ΔP (mercury) = ΔP (air)
ρHg g (h1 −h2) = ρair g mountain
By knowing the density of air, the height of the mountain can be determined and vice versa.
Factors affecting the atmospheric pressure
- Height of the point from the sea level ( inverse proportionality ) where the atmospheric pressure decreases when we go up vertically above sea level due to the decrease in the air column which is causing the pressure.
- Temperature (inverse proportionality).
- Acceleration due to gravity (direct proportionality), Its effect appears in the big heights.
- The density of atmospheric air (The atmospheric pressure increases by increasing the density of air).
Measuring units of atmospheric pressure
The atmospheric pressure can be measured by different units such as cm Hg, atm, Bar, Pascal, and Torr.
Pressure in the required unit = (The quantity to be converted × Atmospheric pressure in the required unit) / Atmospheric pressure in the original unit
Properties of fluids, Factors affecting density and pressure
Applications on pascal’s principle, Manometer types, and uses