Applications on pascal’s principle, Manometer types and uses

The aqueous manometer is preferred to the mercuric one to measure small pressure differences because the density of water is small compared to that of mercury, So, the difference in water levels in the two branches of the manometer becomes clear and easy to measure which decreases the measurement error.

Manometer

The manometer consists of a U-shaped tube containing the proper amount of liquid of known density, One of its ends is connected to the gas reservoir whose pressure is to be measured, and the other end is exposed to air, The pressure at all points in the same horizontal plane in a homogeneous liquid is the same.

A manometer is used in measuring the pressure of enclosed gas, It is used in measuring the difference between the pressure of enclosed gas and the atmospheric pressure.

Manometer types

1. Aqueous manometer: the liquid used is water.
2. Mercuric manometer: the liquid used is mercury.

It is preferable to use the mercuric manometer for measuring high-pressure difference because the mercury has high density, so, mercury neither rushes out the tube nor into the gas reservoir.

How it is used?

If the liquid level in the free end branch is the same liquid level in the other branch that is connected to the gas reservoir, Then, P_a = P_gas  

Δ P = P_gas − P_a = zero

Δ P = zero, If the liquid used is mercury 

If the liquid level in the free end branch is higher than the liquid level in the other branch that is connected to the gas reservoir, Then, P_gas  > P_a.

 P_gas = P_a + ρ g h

Δ P = P_gas − P_a

Δ P = + ρ g h (N / m²)

If the liquid used is mercury then: P_gas = P_a + h

Δ P = P_gas − P_a   ,  Δ P = + h (cm Hg).

If the liquid level in the free end branch is lower than the liquid level in the branch that is connected to the gas reservoir, Then, P_gas < P_a.

P_gas = P_a − ρ g h

Δ P = P_gas − P_a

Δ P = − ρ g h (N / m²)

If the liquid used is mercury then: P_gas = P_a − h

Δ P = P_gas − P_a ,   Δ P = − h (cm Hg)

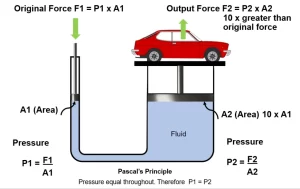
Pascal’s principle

When a liquid is put in a glass container equipped with a piston at the top, then the pressure at point A at depth h is: 

P = P₁ + ρ g h

Where: P₁ is the pressure at the liquid surface and it is due to the atmospheric pressure and the weight of the piston, ρ g h is the pressure of the liquid column on point A, when putting extra load on the piston, the pressure increases by Δ P.

 P = P₁ + ρ g h + Δ P

The piston does not move down because the liquid is incompressible, If the pressure on the piston is increased to a certain limit, the glass container breaks down, This means that the pressure acting on the piston is transferred to all parts of the liquid and to the walls of the container, The French scientist Pascal set his principle based on this result.

Pascal’s principle: When pressure is applied on a liquid in a container, the pressure is transmitted in full to all parts of the liquid as well as the walls of the container, Pascal’s principle is not applied to gases because, unlike liquids, they can be compressed due to the big intermolecular spaces between their molecules.

Applications of Pascal’s principle

1. The hydraulic press
2. The hydraulic brakes of the car
3. The hydraulic lift (It uses a liquid to lift cars in the petrol stations)
   
4. Chair of the dentist
5. The hydraulic drill
6. Diving suit

The hydraulic press

A hydraulic press consists of a tube with 2 pistons at its ends, one small area (a) and the other big area (A) where the space between them is filled with an appropriate liquid such as oil, It is used in lifting high loads using a small force and it is based on Pascal’s principle.

When a force (f) acts on the small piston, pressure (P) is produced, P = f / a, The pressure (P) transfers completely through the liquid to the lower surface of the big piston where a force (F) is produced, P = F / A, In this case the pistons are in equilibrium at one horizontal level where:

Pressure on the small piston = Pressure on the big piston 

P = f / a = F / A

If the force (f) moves the small piston a distance (y₁), then the big piston is affected by a force (F) that moves it a distance (y₂).

Applying the law of conservation of energy (case of ideal press), then:

Work done on the small piston = Work done on the big piston

f y₁ = F y₂

F/ f = y₁ / y₂

Cases of hydraulic press

When the two pistons are at the same level

P = f / a = F / A

When the two pistons are at different levels 

P = (f / a) = (F / A) + ρ g h   or  P = (f / a) + ρ g h = F / A

Where: ρ is the liquid density, h is the difference in height between the two pistons.

The mechanical advantage of the hydraulic press 

The mechanic advantage (η) is determined from the relation:

η =(F / A) = (f / a) = (A / a) = (R² / r²) = (D² / d²) = (y₁ / y₂)= (v₁ / v₂)

Where: R = radius of the big piston, r = radius of the small piston

D = diameter of the big piston, d = diameter of the small piston

v₁ = speed of the small piston, v₂ = speed of the big piston

The mechanic advantage (η) is the ratio between the force produced at the big piston and the acting force at the small piston or it is the ratio between the cross-section area of the big piston to the cross-section area of the small piston.

When the mechanical advantage of a hydraulic press at equilibrium = 400, It means that the ratio between the force produced at the big piston to that acting at the small piston = 400.

The efficiency of the hydraulic press is the ratio between the work done at the big piston to the work done at the small piston, The efficiency of the hydraulic press is determined from the relation: F y₂  ÷ f y₁

When the efficiency of the hydraulic press = 97%, It means that the ratio between the work done at the big piston to the work done at the small piston = 97 / 100.

The efficiency of the hydraulic press does not reach 100 % because of the friction between the piston and the walls of the tube, the gas bubbles in the liquid where work is consumed to reduce the volume of bubbles.

The hydraulic brake system in a car has two types

Drum brake (rear brake): It uses Pascal’s rule as the braking system uses brake fluid, Upon pushing on the brake pedal with a small force and a relatively long stroke (distance), the pressure is transmitted in the master brake cylinder, hence, onto the liquid and the whole hydraulic line, then to the piston of the wheel cylinder outwardly and finally to the brake shoes and the brake drum, A force of friction results in, which eventually stops the car.

Front (disk) brake: In this case, the forces resulting from the braking action press on the brake pads which produce friction enough to stop the wheel, It should be noted that the distance travelled by the brake shoes in both cases is small because the force is large.

Diving suit

It protects the diver from the high pressure in the very deep seas where the air is blown inside it to protect the head of the driver from the high pressure.

Applications on the pressure at a point (Connected vessels, U-shaped tube, and Mercuric barometer)

Properties of fluids, Factors affecting density and pressure

Steady flow, Turbulent flow, and Applications on the continuity equation