Acceleration types, units, importance and Graphic representation of moving in a straight line

When the driver of a moving car uses the brakes, we describe the car’s movement as a decelerating motion because the car’s speed decreases as time passes. The ratio d/t remains constant in the case of a body that moves at a uniform speed because this body covers equal distances at equal periods. The body that moves at acceleration can’t move at a regular speed because its speed changes by passing time.

Graphic representation of moving in a straight line

Graphs and tables are important to physicists, Physical phenomena can be described and understood in many ways, Physicists use mathematical relations between variables to describe certain phenomena. Physicists use other mathematical relations like graphs and tables to:

• predict the relation between certain physical quantities.
• understand practical results.
• describe the physical phenomena more easily.

Graphic representation of regular (uniform) speed

Facts about regular speed in a straight line:

1. The (distance – time) graph of a regular motion at a constant (uniform) speed is represented by a straight line passing through the origin point because the distance is directly proportional to the time, when the object moves at a constant speed.
   
2. The (speed-time) graph of a regular motion at a constant (uniform) speed is represented by a straight line parallel to the time axis.

The (distance- times) graph of an object at rest is represented by a straight line parallel to the time axis. The relation (distance- time) graph for an object that moves at a non-uniform speed is represented as a curved line passing by the origin point.

Acceleration

When you sit in a car next to the driver and the car starts moving from rest in a straight line, you notice that the car speed increases gradually as time passes. When the speed of the car at 1 second becomes 5 m/sec., at 2 seconds becomes 10 m/sec., at 3 seconds becomes 15 m/sec. and so on.

To describe the change in the car speed in one second in this case, we use a physical quantity called “acceleration“. Acceleration is the change of an object speed in one second in a specific direction, or it is the rate of change of speed.

Acceleration (a) = Change in speed (ΔV)/Time in which the change occurs (Δt)

Acceleration (a) = [Final speed (V₂) − Initial speed (V₁)]/ Time (Δt)

Delta (Δ) means the rate of change of any physical quantity.

To calculate V₁ or V₂:    V₁ = V₂ − (a × t)      ,    V₂ = V₁ + (a × t)

Measuring units of acceleration 

Acceleration unit = metre/second². We use the acceleration unit (m/sec².) when the speed is measured in metre/second and the time is measured in second, or (km/h²) when the speed is measured in kilometre/hour and the time is measured in hour.

Guidelines to solve problems on acceleration:

1. To calculate: Acceleration, Change in speed, and Time, a = ΔV/ Δt
2. If the body is moving at a regular speed, so its acceleration equals zero because its speed doesn’t change as time passes [when (AV) = Zero, then (a) = Zero].
3. If the body starts moving from rest, so its initial speed (V₁) equals zero.
4. When the body stops moving, so its final speed (V₂) equals zero.
5. When the car is moving, then the brake is applied to stop the car after a period of time, so, its final speed equals zero.

Uniform acceleration

When a car covers equal distances at equal periods, it is said that the car moves at a uniform (regular) speed. But if its speed changes (decreases or increases) by equal values at equal periods, it is said that the car moves at a uniform (regular) acceleration.

When the speed of the car increases by 10 m/sec each 2 sec, so, Acceleration (a) = (20- 10)/ 10 = 5 m/sec². Uniform acceleration is an acceleration by which an object moves in a straight line when its speed changes by equal values through equal periods.

When a car moves at a uniform acceleration equals 5 m/sec². This means that the car moves in a straight line and its speed changes with 5 m/sec. each one second.

Types of uniform acceleration

1. Positive acceleration.
2. Negative acceleration.

Positive acceleration

The object moves at a uniform positive acceleration (accelerating motion), when [Its initial speed < Its final speed]. Positive acceleration is an acceleration by which an object moves in a straight line when its speed increases by equal values through equal periods.

When an object moves at a positive acceleration equals 2 m/sec². This means that the object moves in a straight line and its speed increases by 2 m/sec, each one second. The sign (+) refers that the speed of the object increases regularly by 2 m/sec. each one second.

Negative acceleration

The object moves at a uniform negative acceleration (decelerating motion). [Its initial speed > Its final speed]. Negative acceleration is an acceleration by which an object moves in a straight line when its speed decreases by equal values through equal periods

When an object moves at a negative acceleration equals -2 m/sec². This means that the object moves in a straight line and its speed decreases by 2 m/sec each one second. The sign (−) refers that the speed of the object decreases regularly by 2 m/sec. each one second.

So, we can deduce the type of acceleration from the shape of the graph:

1. Positive acceleration: when the object moves at (a non-uniform speed), V (initial) < V (final), So, it moves at an accelerating motion.
   
2. Negative acceleration: The object moves at (a non-uniform speed), V (initial) > V (final), So, it moves at a decelerating motion.
   
3. Zero acceleration: The object moves at (a uniform speed), V (initial) = V (final).
   

The acceleration is positive when its value increases, while it is negative when its value decreases because when moving with a positive acceleration, the final speed is greater than the initial speed, while when moving with negative acceleration, the final speed is less than the initial speed.

The object which moves at a uniform speed, its acceleration equals zero. A body moves at zero acceleration because its speed doesn’t change by passing time (ΔV = Zero).

Problems

• An object moves from rest and its speed reaches 20 m/sec. in 5 seconds. Calculate the acceleration of the moving object. What is the type of acceleration?

Solution

V₁ = 0   , V₂ = 20 m/sec.  , Δt = 5 sec.

a = V₂ – V₁ /Δt

a = (20- 0)/5= 4 m/sec² , Positive acceleration.

• A train moves at a uniform speed of 20 m/sec. When the driver uses the brakes, the train stops after 4 sec. Calculate the acceleration at which the train moves and mention the type of acceleration.

Solution

V₁ = 20 m/sec.  V₂ = 0  ,  Δt = 4 sec

a = V₂ – V₁ /Δt

a= (0 – 20)/4 = – 5 m/sec²   , It’s decelerating motion

• A car driver moves at a speed of 80 m/sec. used the brakes to make the car move at a uniform decreasing acceleration 2 m/sec? Find the car speed after 12 seconds of using the brakes.

Solution

V₁ = 80 m/sec , V₂=56 m/sec  , a = – 2 m/sec  , Δt = 12 sec

V₂ = V₁ + (a × t)

V₂ = 80 + ( – 2 × 12) = 56 m/sec

Guidelines to solve problems

On moving at a uniform speed through the period (AB) and then followed by the movement at a uniform acceleration through another period (BC). So, The uniform speed through the period (AB) = The initial speed through the period (BC). [V₁ at point (B)].

• An object moves in a straight line at a regular speed. If the time taken by the object to move from (A) to (B) is 4 seconds, then it moves at a uniform acceleration from point (B) until it stops at point (C) in 20 seconds. Calculate each of the following:

1. The regular speed of the object to cover the distance (AB).
2. The uniform acceleration by which the object moves from point (B) to point (C).

Solution

1. Regular speed at (AB) = Distance/Time = 40/4= 10 m/sec.

2. The initial speed at (BC) = The uniform speed at (AB) = 10 m/sec

The uniform acceleration at (BC) (a) = (V₂ – V₁)/At = (0 – 10) /20 = – 0.5 m/sec²

• A car moves at a speed of 60 m/sec., if the driver used the brakes to decrease the speed by 3 m/sec. Calculate the time after which the car stops.

Solution

V₁ = 60 m/sec, V₂ = 0 , a = – 3 m/sec² ,   Δt = ?

a = (V₂ – V₁)/ Δt

Δt = V₂ – V₁/ a

a = (0 – 60)/- 3

a = 20 sec

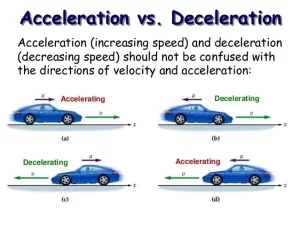
What is the difference between acceleration and deceleration?

Acceleration and deceleration are both terms used to describe changes in the motion of an object, but they refer to opposite changes. Both acceleration and deceleration are measured in units of meters per second squared (m/s²). A positive value indicates acceleration, while a negative value indicates deceleration.

Acceleration is the rate at which an object’s velocity changes. Velocity considers both the speed and direction of the object. So, acceleration can describe an object speeding up or changing direction.

• If the speed is increasing, we call it positive acceleration.
• If the object is slowing down or changing direction (even if it ends up speeding up in a different direction), we call it negative acceleration.

Deceleration is a specific type of acceleration. It refers to when the object’s speed is decreasing. In other words, it’s negative acceleration. Deceleration is also sometimes called slowing down or retardation.

Think about acceleration like the gas pedal in a car. Pressing down increases the speed (positive acceleration), and letting off the pedal (or even applying the brakes) decreases the speed (negative acceleration or deceleration).

Some everyday examples:

• A car speeding up from a stoplight is accelerating (positive).
• A bike coming to a stop sign is decelerating.
• A roller coaster going up a hill is accelerating (negative), even though its speed is decreasing.

You can subscribe to Science Online on YouTube from this link: Science Online  

You can download the Science Online application on Google Play from this link: Science Online Apps on Google Play

Physical Quantities, Scalars, Vectors, Distance, Displacement, Speed & Velocity

Motion in one direction, Types of Speed, Average Speed & Relative Speed

Types of motion, Relative motion, Applications of Mechanical waves & Electromagnetic waves

Role of waves in transferring energy, Wave Motion, Transverse waves & Longitudinal waves

Laws of circular motion (Centripetal Acceleration, Tangential linear Velocity & Centripetal Force)