Steady flow, Turbulent flow and Applications on the continuity equation

We can distinguish between two types of flow in fluids which are Steady flow and Turbulent flow, When a liquid moves such that its adjacent layers slide smoothly with respect to each other, we describe this motion as a laminar flow or a streamlined (steady) flow, Every small amount of the liquid follow a continuous path called streamline.

Steady flow

Steady flow is the flow at a low speed such that its adjacent layers slide smoothly with respect to each other, Streamline is an imaginary line that shows the path of any part of the fluid during its steady flow inside the tube, The density of the streamlines at a point is the number of streamlines crossing perpendicular a unit area point.

Characteristics of the streamlines

1. Imaginary lines do not intersect.
2. The tangent at any point along the streamline determines the direction of the instantaneous velocity of each particle of the liquid at that point .
   
3. The number of streamlines does not change as the cross-section area changes, while the streamlines density at a point changes as the cross-section area changes and expresses the flow velocity of the liquid at that point.
4. Therefore, streamlines cram up at points of high velocity (its density increases) and keep apart at points of low velocity (its density decreases), This means that the speed of the fluid at any point inside the tube is directly proportional to the density of streamlines at that point.

Conditions of the steady flow

1. The liquid should fill the tube completely.
2. The speed of the liquid at a certain point in the tube is constant and does not change as time passes.
3. Flow is irrotational, there is no vertex motion.
4. No frictional forces between the layers of the nonviscous liquid.
5. Flow such that the amount of liquid entering the tube equals that emerging out of it in the same period because the liquid is incompressible.

Flow rate is the quantity of liquid flowing through a certain cross-sectional area of a tube in one second, Flow rate could be volume flow rate and mass flow rate.

Volume flow rate (Q_v) is the volume of fluid flowing through a certain area in one second, the measuring unit is m³/s, When the volume rate of a liquid = 0.05 m³/s, It means that the volume of fluid flowing through a certain area in one second = 0.05 m³.

Mass flow rate ( Q_m ) is the mass of fluid flowing through a certain area in one second, The measuring unit is kg/s, when the mass flow rate of a liquid = 3 kg/s, It means that the mass of fluid flowing through a certain area in one second = 3 kg.

Calculating the flow rate at any cross-sectional area:

Considering a quantity of liquid of density (ρ), volume (V_ol), and mass (m) flowing in speed (v) to move a distance (Δx) in time (Δt) through the cross-sectional area of the tube (A).

From the definition of the volume flow rate:

Q_v = ΔV_ol / Δt

ΔV_ol = A Δx = A v Δt  , where Δx = v Δt  

∴ Q_v = ( A v Δt ) / Δt

Q_v = A v

From the definition of the mas flow rate:

Q_m = Δm / Δt

Δm = ρ ΔV_ol

ΔV_ol = A Δx = A v Δt

Q_m = ( ρ A v Δt  ) / Δt

Q_m = ρ A v = ρ Q_v

The amount of liquid entering the tube = that emerging out of it in the same period.

Flow rate (volume or mass) is constant at any cross-sectional area and this is called the law of conservation of mass that leads to the continuity equation.

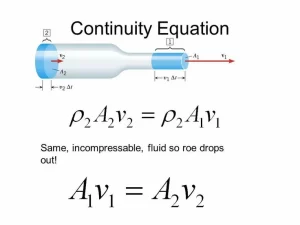
Deduction of the continuity equation (relation between flow speed of liquid and cross-sectional area of the tube) 

Imagine that a tube has a fluid in a steady flow where the previous conditions of steady flow are verified.

Consider two-cross sectional areas (A₁ , A₂) perpendicular to the streamlines:

At first cross-sectional area (A₁), the speed of liquid through it (v₁) then :

The volume flow rate :  Q_v = A₁ v₁ , The mass flow rate: Q_m = ρ A₁ v₁

At the second cross-sectional area (A₂), the speed of liquid through it (v₂) then:

The volume flow rate: Q_v = A₂ v₂, the mass flow rate: Q_m = ρ A₂ v₂

The flow rate (volume or mass) is constant in case of steady flow.

 ρ A₁ v₁ = ρ A₂ v₂

A₁ v₁ = A₂ v₂

 v₁ / v₂ = A₂ / A₁, this relation is called the continuity equation 

The continuity equation

The velocity of a fluid in a steady flow at any point is inversely proportional to the cross-sectional area of the tube at that point.

Based on the previous relation (A₁ v₁ = A₂ v₂) if:

The tube is cylindrical having two cross-sectional areas one is wide and the other narrow.

A₁ v₁ = A₂ v₂

r²₁ v₁ = r²₂ v₂

The tube is branched into ( n ) branches of the same cross-sectional area.

A₁ v₁ = n A₂ v₂

r²₁ v₁ = n r²₂ v₂

The tube is branched into several branches of different cross-sectional area 

A₁ v₁ = A₂ v₂ + A₃ v₃ + A₄ v₄

r²₁ v₁ = r²₂ v₂ + r²₃ v₃ + r²₄ v₄

Where: A = π r², r = radius of the tube.

The speed is inversely proportional to the cross-sectional area (v ∝ 1/A), so, the liquid flows slowly in the tube when its cross-sectional area is big and vice versa.

Applications on the continuity equation

Flow of blood is faster in the main artery than in the blood capillaries because the sum of cross-sectional areas of blood capillaries is greater than the cross-sectional area of the main artery and since ( v ∝ 1/A ), so, the speed of blood decreases in the blood capillaries to allow the exchange of oxygen and carbon dioxide gases in the tissues to supply it with food.

Design of the gas opening in the stoves, Opening are small so that the gas rushes fast out of it at a high speed (v ∝ 1/A).

Turbulent flow

The turbulent flow is the flow when the speed of the fluid exceeds a certain limit and is characterized by small eddy currents, The steady flow of a fluid ( liquid or gas ) becomes turbulent flow if :

1. The speed of the fluid exceeded a certain limit and is characterized by small eddy currents.
2. A gas transfers from a small space to a wider space.
3. A gas becomes turbulent when it transfers from high pressure to low pressure.

Applications on the pressure at a point (Connected vessels, U-shaped tube & Mercuric barometer)

Applications on pascal’s principle, Manometer types and uses

Factors affecting the force of viscosity and Applications on the viscosity